Maybe I should begin by stating that "the summit issue is reflecting how IDW models the surface, rather than weighting". IDW sees the predicted surface as an averaging model, while Spline tries to minimize abrupt change to make 'smooth rubber sheet' and Kriging tries to minimize errors. (I hope this makes my point clear).
Let me focus on the difference between surfaces predicted by IDW and Kriging, especially how they are related to measured data (without mathematics).
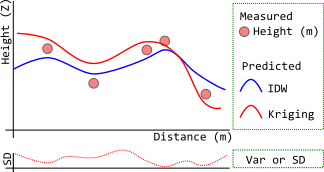
Above (my poor drawing) is trying to picture that:
- IDW (blue line) estimates the values as averages of measured samples, and its surface will not pass through sample points. Its smoothness is controlled by power value, but even bumpy - high power settings cannot make it above the maximum or below the minimum (so, yes, summit problem). Because power is merely working as relative influence of each sample point.
- Kriging, like Spline, can account general trends and estimate beyond range of sample data. On the above example, though not obvious, measured height has ana mild increase toward left-hand side... where Kriging (red line) surface goes higher than the sample points. The surface pass through sampling points if the variance (or s.d.) is zero, but not necessary.
I did not answer the weighting aspect of the question, the subject we cannot go without mathematics... and I am really bad at it.