It is basically possible to do what you want, but not the easy way.
I did the following with QGIS:
Restricting the manipulation thto the northern hemisphere, I clipped the Natural Earth's land polygon and degree grid to positive values of latitude. Then I created a custom CRS for laea projection centered on the North Pole:
+proj=laea +lat_0=90 +lon_0=0 +x_0=0 +y_0=0 +ellps=GRS80 +towgs84=0,0,0,0,0,0,0 +units=m +no_defs
and reprojected the data to it.
In a second step, I created a similiar laea projection at 0°E/0°N:
+proj=laea +lat_0=0 +lon_0=0 +x_0=0 +y_0=0 +ellps=GRS80 +towgs84=0,0,0,0,0,0,0 +units=m +no_defs
and assigned it to the data from the last step. Note that I did not reproject the data to the new CRS in this step!
Then I reprojected that dataset back to WGS84, which puts the North pole into the middle of the Atlantic.
This is the picture I got:
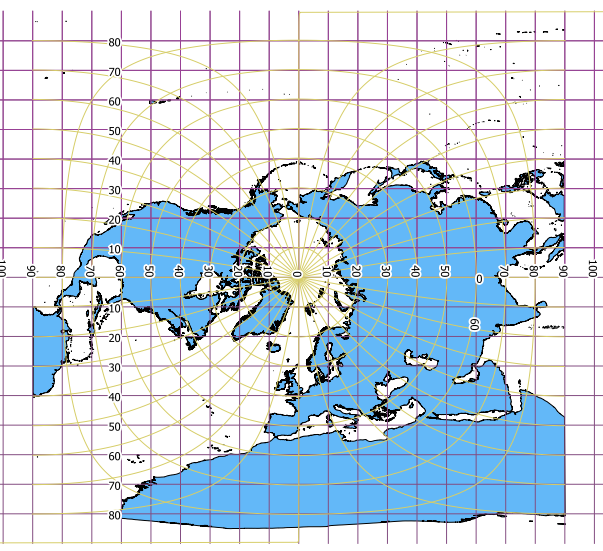
I included the new degree grid in purple and the traditional grid in light green. The result is obviously useless around the equator, but should be usefull around the north pole.
EDIT
A very simple reprojection from Greenwich coordinates to North pole coordinates using a sphere would be:
En = (90°-Ng) * sin (Eg/180° * PI())
Nn = (Ng-90°) * cos (Eg/180° * PI())
With Eg and Ng degrees East and North in Greenwich coordinates (EPSG:4326) En and Nn degrees of a geographic Coordiante system centered at the north pole.
This will give you concentric circles for the Greenwich latitudes.
