What's the purpose of the reference latitude in Lambert Conformal Conic?

Question

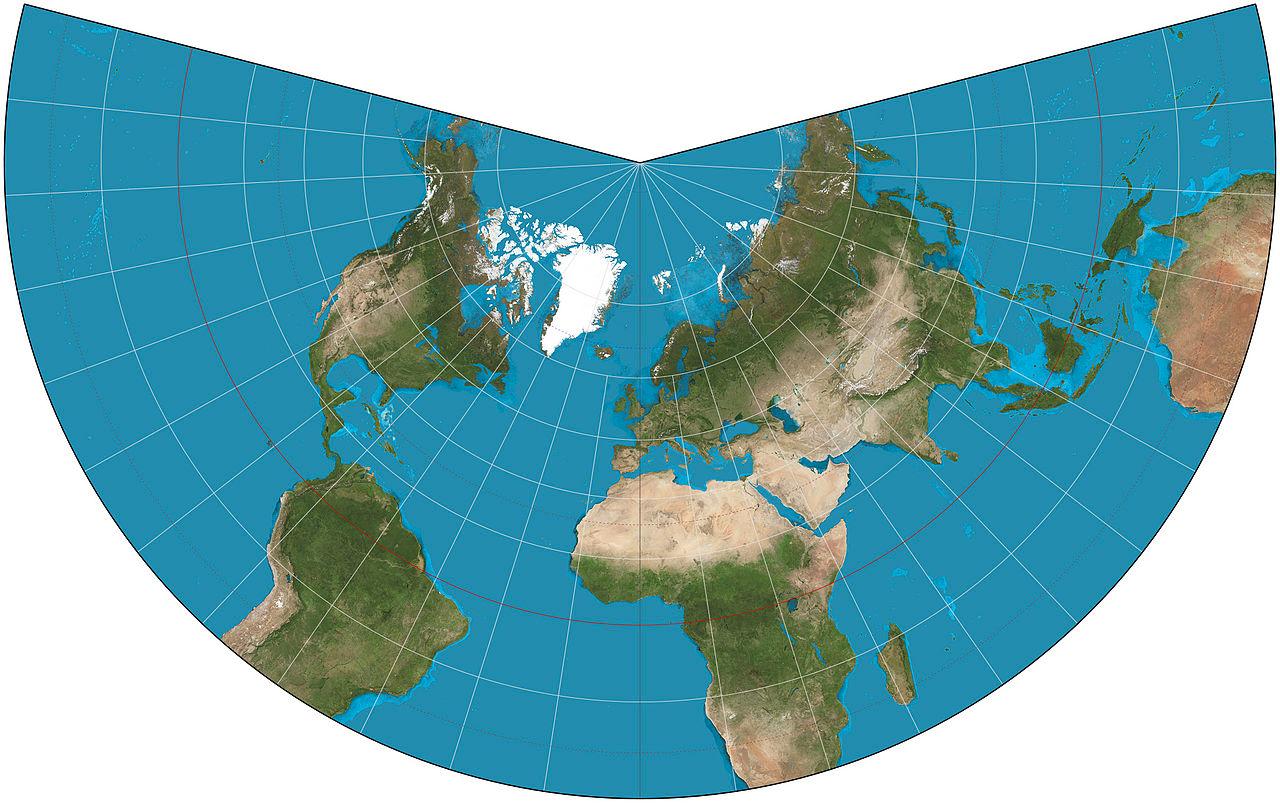
Going through flight school I got acquainted with the Lambert Conformal Conic projection, as it's the common projection for VFR sectional charts, and I think I have pretty good grasp of it, geometrically.

However, trying to wrap my head around the mathematics behind it I browse around on Wikipedia and on MathWorld which both more or less simply list the formulas, not really diving into the mathematics behind it.

It appears to have 4 parameters:

• The two standard parallels,
• reference longitude, and reference latitude

What is the purpose of the reference longitude/latitude? Do they affect the actual projection, or will they simply shift the result to let the reference coordinates result in (x,y)=(0,0)?

Answer 1

I think you have it "spot on" so to speak.

Fundamental to the geometry of any projection is the standard line or lines -- where the "paper" touches or cuts the globe before being "rolled flat" and where there is no linear distortion.

Then the practical issue is where to center the map, if it's not at 0, 0 (off the Gulf of Guinea): The center of your region of interest becomes the reference lat-long.

For a conic projection, the effect of the reference longitude will be to rotate your region of interest round to a prominent position. The map below (from wiki/Lambert_conformal_conic_projection) uses the default reference meridian, but if you wanted some other meridian to be "vertical"...

Answer 2

The choice of the reference lat/long does not create a simple shift. This shift in the XY coordinate system can be done using false easting and northing.

The reference meridian is the center of the developed plane. It will be the only meridian that is parallel to the Y axis. So if you change the meridian of reference, you will shift and rotate your coordinate system.

The information of the reference parallel is not necessary because it is the average of the two standard parallels. In fact you can also define the projection based on the latitude of the reference parallel (which correspond to the angle at the top of the cone) and a scale factor (With a scale factor of one, your projection is tangent).

Note that the latitude of origin, which defines the position of the origin of the coordinate system, is not always the same as the latitude of reference.