Topographic Index - Why is upslope contributing area divided by downslope contour width and not upslope contour width?

Question

In the Topographic Index calculation, a is the upslope contributing area per unit contour length. Is the "unit contour length" calculated from examining the contour widths of the downslope cells that water in the current cell flows to? Or is it the contour widths of the upslope cells that flow to the current cell?

In Quinn and Beven's work, it seems to be the the downslope cells. Why? How does that make more hydrological sense than using the upslope cells' contour widths? Can some justify the use of the downslope (from the current cell) cells' contour widths vs the upslope (from the current cell) cells' contour widths for me?

Edit - Hopefully these pictures and this description provide the details people are looking for.

Let's examine a 3 x 3 grid with elevation values and a 10m cell size resolution. Let's assume we are working with a MFD like algorithm. The grid is pictured below:

In this grid, the center cell, with a value of 5, receives flow (and therefore area) from the upslope cells with higher elevation values. So it receives flow (and therefore area) from the cells that contain elevation values of 8, 7, and 6. Different algorithms (Quinn, Freeman, etc.) can dictate how much area the upslope cells pass to the center cell based on the fraction that particular slope (upslope cell to center cell) contributes to the sum of all the slopes (upslope cell to all downslope cells). Anyway, however it is decided, accumulated area ends up in the center cell passed to it from its upslope neighbors. This accumulated area can also be called the upslope contributing area.

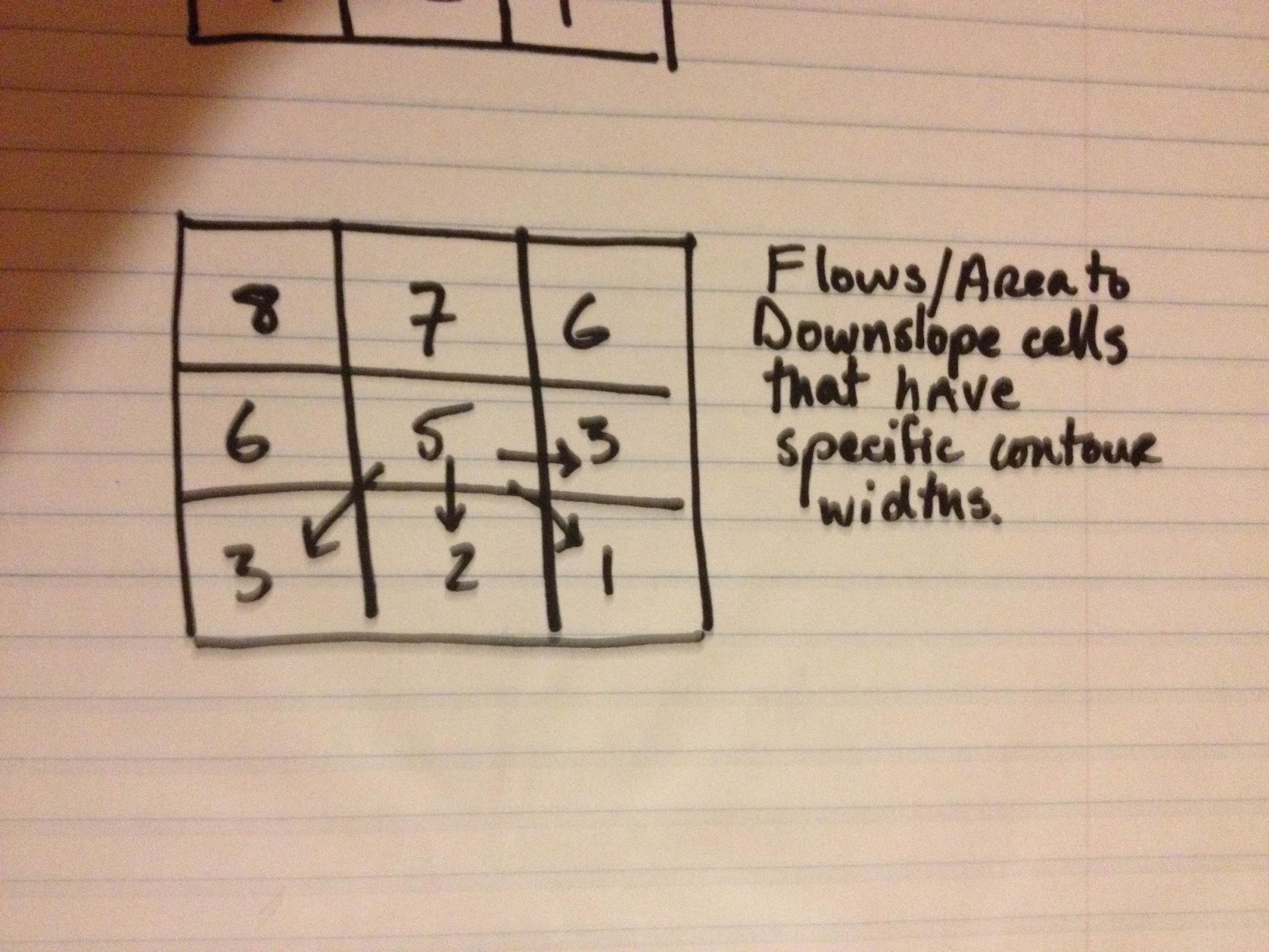
In this image we see the downslope cells that the center cell passes area/flows into. Now in Quinn's description of his algorithm, he says :

Thus the actual value of a/tan ß for the current cell will be the total area draining through that cell acting across a contour length equal to the sum of the downhill contour lengths and divided by the weighted average tan ß value.....(THE PREDICTION OF HILLSLOPE FLOW PATHS FOR DISTRIBUTED HYDROLOGICAL MODELLING USING DIGITAL TERRAIN MODELS)

What I am confused about is this:

Since the upslope contributing area is dictated by the both the slope between the upslope cells and the center cell as well as contour width determined by the direction (cardinal vs. diagonal), why are the downslope contour widths used? The upslope contributing area per unit contour width, the numerator in the TWI/TI, seems to dictate how wet an area will be due to accumulated flow from upslope cells - why divide it by the the contour widths of the flows being passed downslope? And the denominator seems to get at how wet a cell will be in relation to it's steepness, with the steeper the drop to the downslope cells the larger the sum of their slopes, and the larger the denominator will be and the lower the overall TI value will be.

The denominator I understand fine, but I can't seem to wrap my brain around why the area passed into the cell is divided by the downslope contour widths....and not the contour widths of the flows entering the center cell from the upslope cells.

Answer 1

When you perform a flow accumulation on a DEM, the upslope area is defined as all of the inflowing grid cells AND the cell itself. Thus, a grid cell is actually part of its own contributing area. The minimum upslope area (not specific contributing area) is equal to the area of one grid cell, i.e. a grid cell with no inflow cells. I think this is the reason that we specific contributing area is defined as the upslope area divided by the downslope contour length.