Spherical geodesics
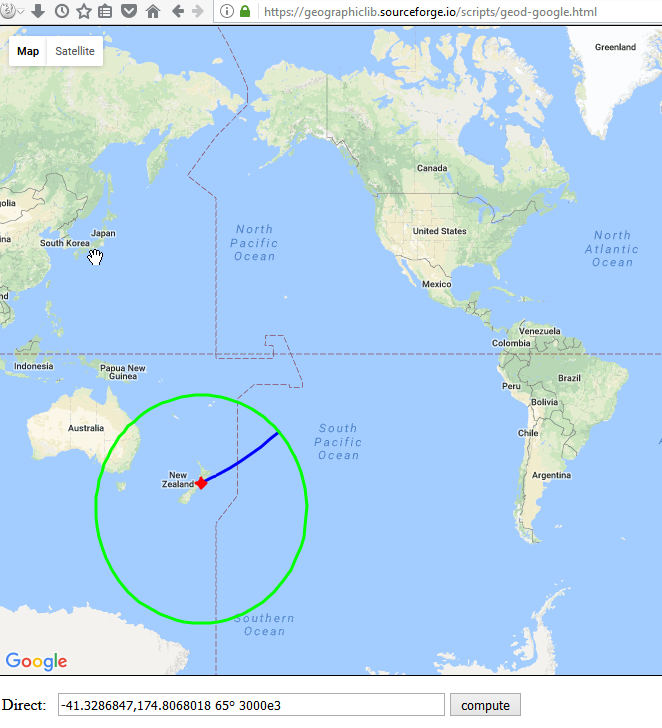
But let's take a step back. If we first assume earth is a perfect sphere, then we can select any point (A) on that surface, and draw a geodesic to some radial distance, R, to make a big circle (with radius R) on that sphere. As we increase the radius to more than 1/4 way around the sphere, the circumference of the the circle will become larger than the "circumference of the sphere" which is its diameter. Now, if we start looking at the sphere from the other side, that of the diametrically opposite of point A, which we call point B. We see that radius (approaching us) is still a circle, but now shrinking. (Imagine a rubber band being stretched around a ball to the other side.)
Ellipsoidal geodesics
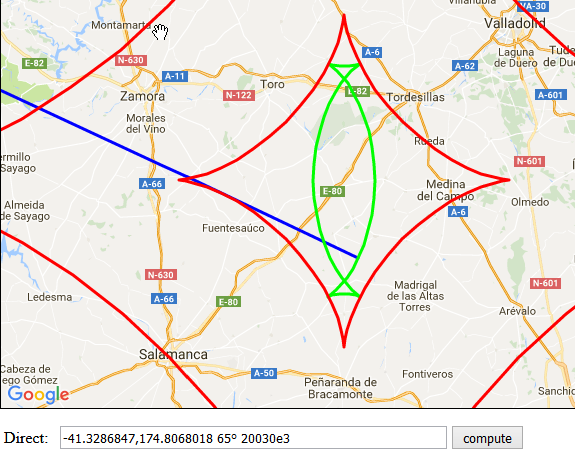
However, Earth is actually an ellipsoid, which in its simplest form, is a a sphere that has been flattened on its poles. Therefore, a geodesic radius radius used to define a "circle" will almost never actually look like a circle circle, unless its center is at one of the poles. That is because the geodesic geodesic distance changes depending on which direction (bearing) you draw draw the radius, except at the polar symmetry axis. The two things to note are:
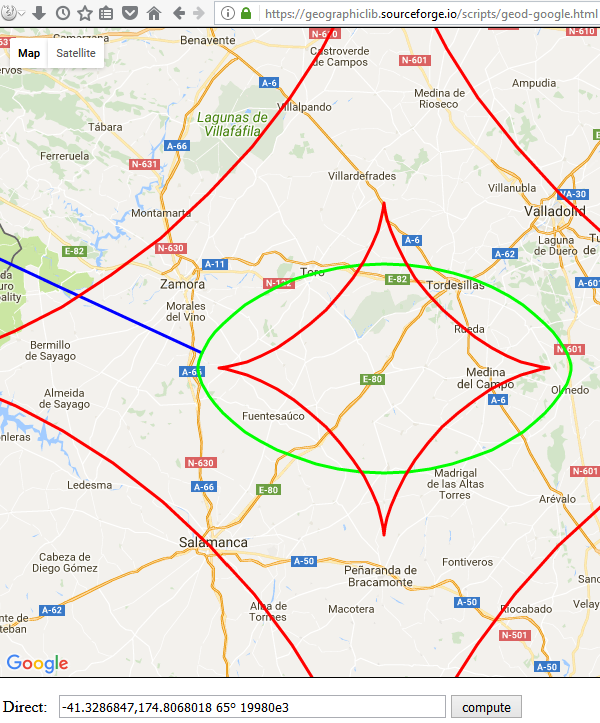
As we progress through the picture series we see that, as the radius (in
green green) is getting closer to point B, the circle is getting more and more
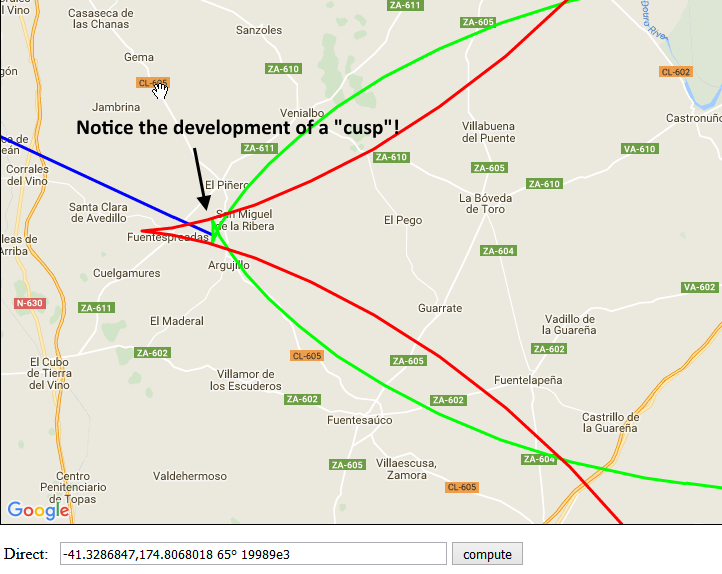
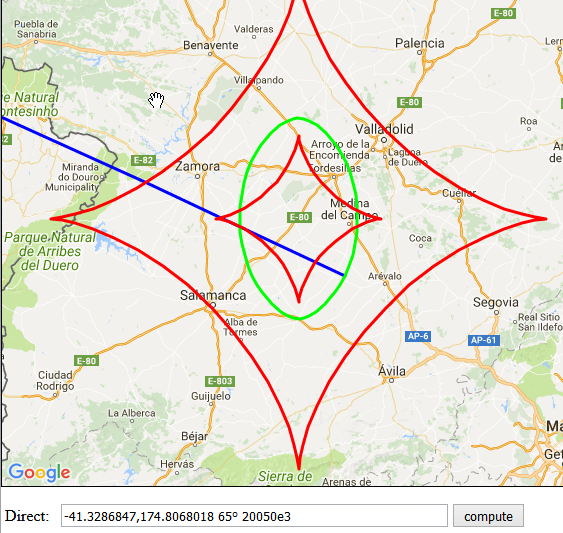
distorted distorted. At the point when it first reaches the inner envelope (in
red red), it develops a cusp. This cusp changes shape until the radius
exits exits the other side of inner envelope. At that point, it once again
resembles resembles the shape of a distorted circle or ellipse. Mathematically, I
suppose suppose this is equivalent to having inverted the circle.
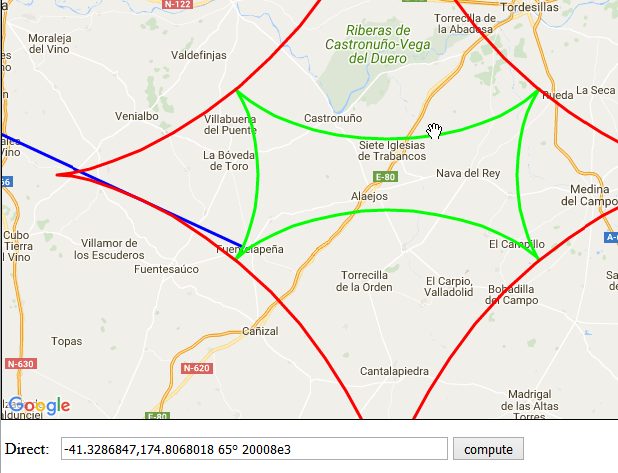
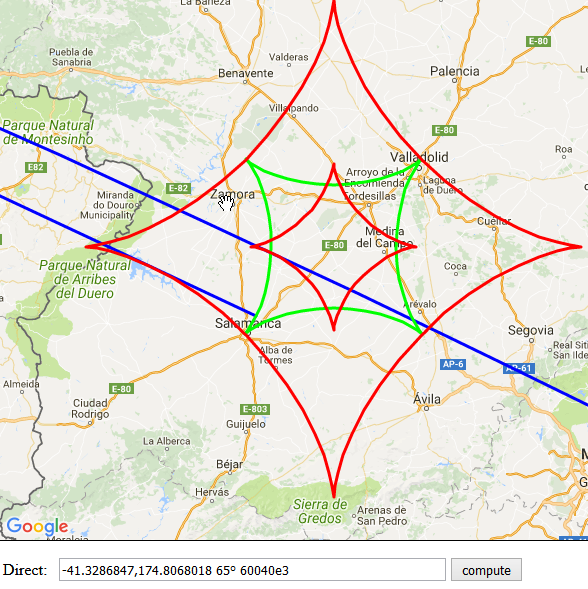
In the last picture, we have increased the radius to loop around the
planet planet, one more time, to end up near B. However, now see the meaning
of of the envelope. Because ellipsoidal geodesics are not closed loops,
the the envelope astroids represent the geodesic difference between each non-closed loop.