Since posting my question, I have made some progress in better understanding the use of the envelope. To illustrate my findings, let's first consider what happens to the concept of a geodesic radius in the two cases of (1) a Sphere, and (2) an 1-Ellipsoid. (I call it a "1-ellipsoid" as it is a spheroid only distorted along one axis and not both. For distortions along both axes, I would call it a 2-Ellipsoid.)
Working Example
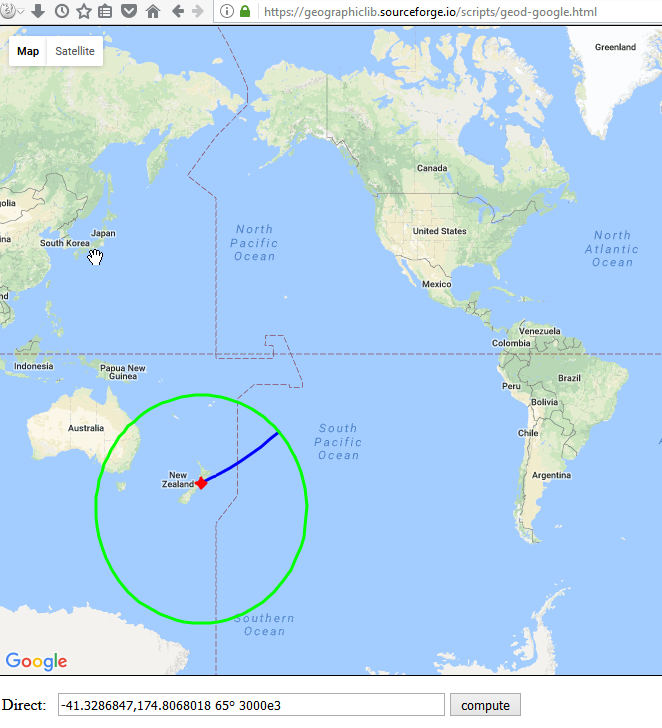
As a starting point (A) we chose Wellington (New Zealand) and try to find it's approximate geographic opposite (B) on the planet, we will end up slightly outside Salamanca (Spain).
(A) Wellington: -41.3286847, 174.8068018
(B) Salamanca (Alaejos): 41.3046662, -5.1947402
Here we will use the direct problem to find an approximate location for B. The inverse problem would find the exact position.
Spherical geodesics
But let's take a step back. If we first assume earth is a perfect sphere, then we can select any point (A) on that surface, and draw a geodesic to some radial distance, R, to make a big circle (with radius R) on that sphere. As we increase the radius to more than 1/4 way around the sphere, the circumference of the the circle will become larger than the "circumference of the sphere" which is its diameter. Now, if we start looking at the sphere from the other side, that of the diametrically opposite of point A, which we call point B. We see that radius (approaching us) is still a circle, but now shrinking. (Imagine a rubber band being stretched around a ball to the other side.)
There are two facts to observe in this case:
- Drawing any geodesic of any length >PiR will always pass through the opposite "pole".
- The geodesic is a closed circle.
Ellipsoidal geodesics
However, Earth is actually an ellipsoid, which in its simplest form, is a sphere that has been flattened on its poles. Therefore, a geodesic radius used to define a "circle" will almost never actually look like a circle, unless its center is at one of the poles. That is because the geodesic distance changes depending on which direction (bearing) you draw the radius, except at the polar symmetry axis. The two things to note are:
- Geodesics are not closed paths
- The mathematical circles generated by the geodesic radius are not Cartesian circles
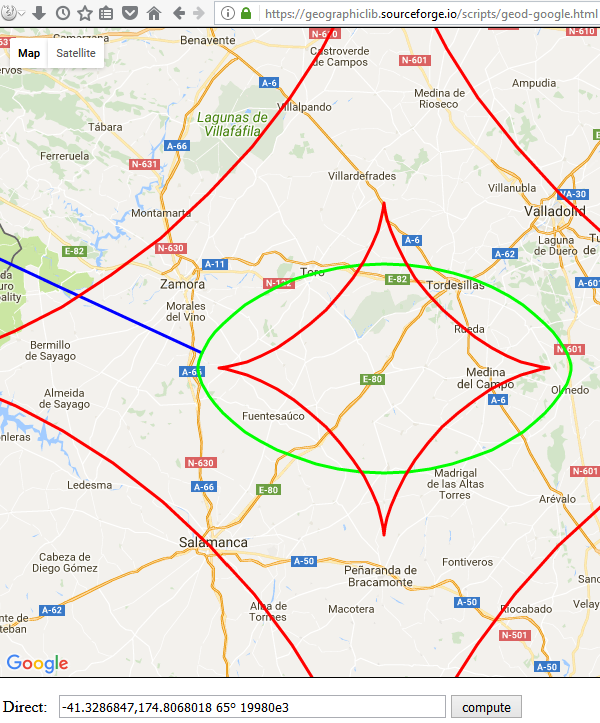
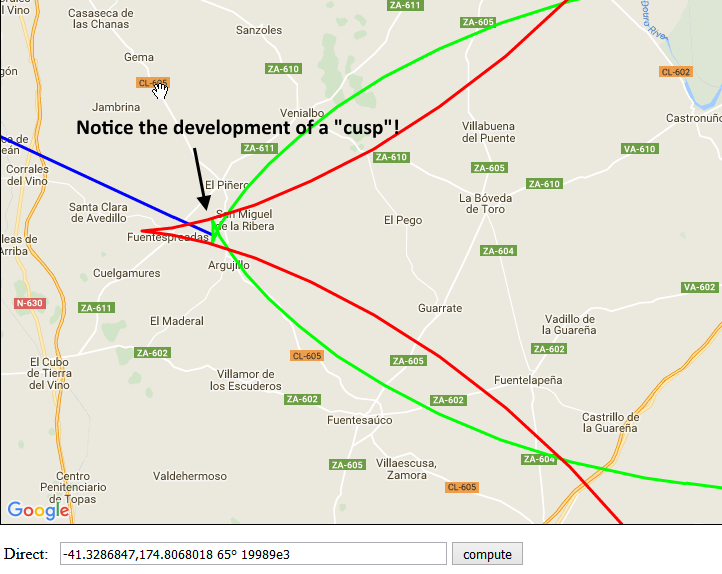
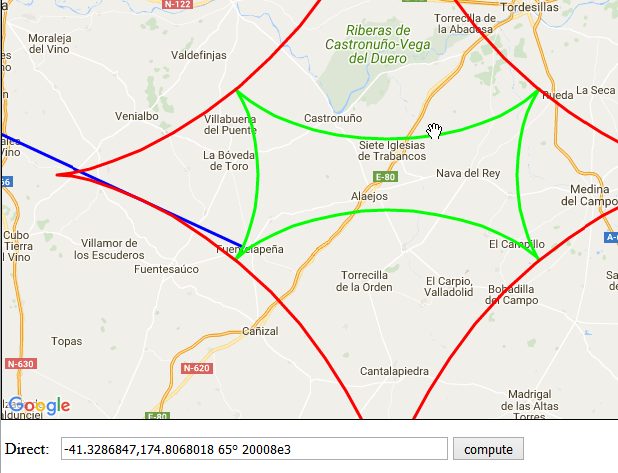
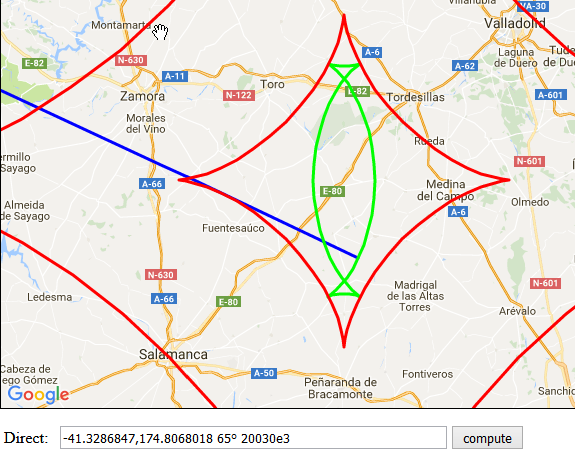
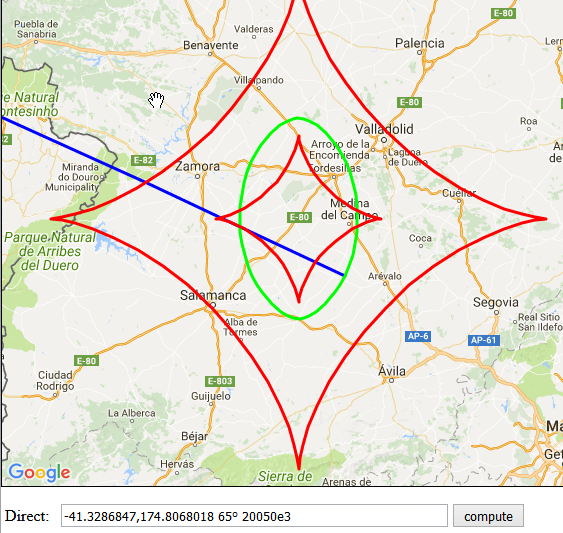
Looking at the following picture series of geodesic radii, we find that the radial "circle" changes shape as it expands around the ellipsoid. Of course mathematically it is always a perfect circle, but by human perception, it is distorted from our flat world experience.
As we progress through the picture series we see that, as the radius (in green) is getting closer to point B, the circle is getting more and more distorted. At the point when it first reaches the inner envelope (in red), it develops a cusp. This cusp changes shape until the radius exits the other side of inner envelope. At that point, it once again resembles the shape of a distorted circle or ellipse. Mathematically, I suppose this is equivalent to having inverted the circle.
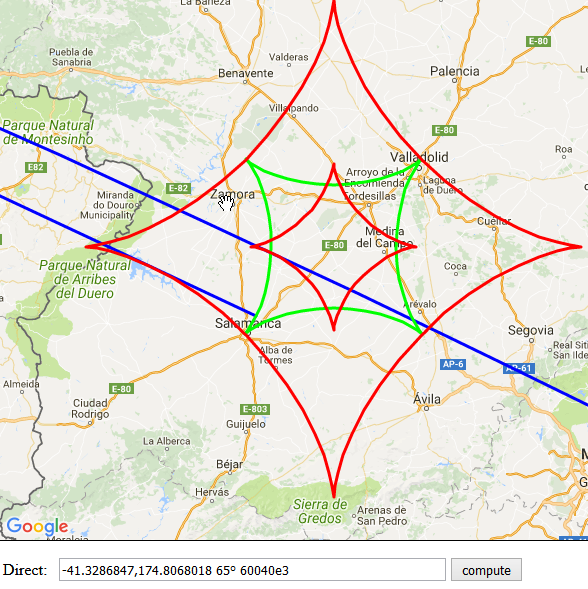
In the last picture, we have increased the radius to loop around the planet, one more time, to end up near B. However, now see the meaning of the envelope. Because ellipsoidal geodesics are not closed loops, the envelope astroids represent the geodesic difference between each non-closed loop.
I'm sure someone else can write and make a much better explanation, so please feel free to comment and revise.