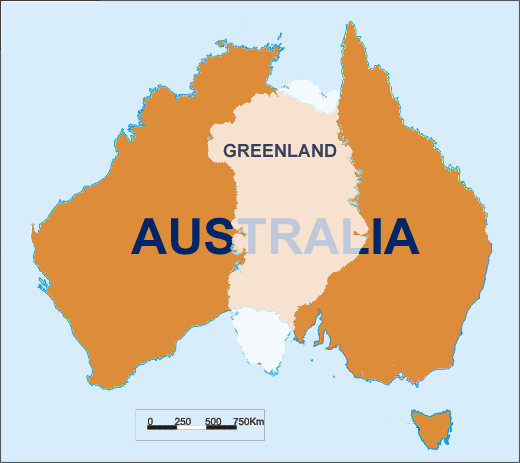
The Mercator projection increases scale along with the latitude. Greenland seems larger than Australia, when in fact it is much smaller. The equal-area projections however stretch shapes away from the Equator. I suppose that there exist no projection that preserves size and shape at the same time. But for the purpose of comparing countries it is in fact not needed to preserve any properties exactly. The distortions should be only roughly the same in any point of the map. What is the map projection that accomplishes it most closely?