Creating radial flow diagram for multiple lines with same start and end point using ArcGIS Pro

Question

I have a dataset of start points and end points that I would like to connect. I used "XY to line" tool in ArcGIS Pro. Using this approach, records that have same start and end point will overlap, and show as one line. However, my goal is to show all the records as different lines as shown in the figure.

Is there any way to do that in ArcGIS pro?

Answer 1

This is nice geometry exercise and requires coding. To create a family of curves we can compute deviation from straight line using 2 randomly selected parameters; use parabola for smooth transition; draw variable length perpendiculars to actual line and connect their end points:

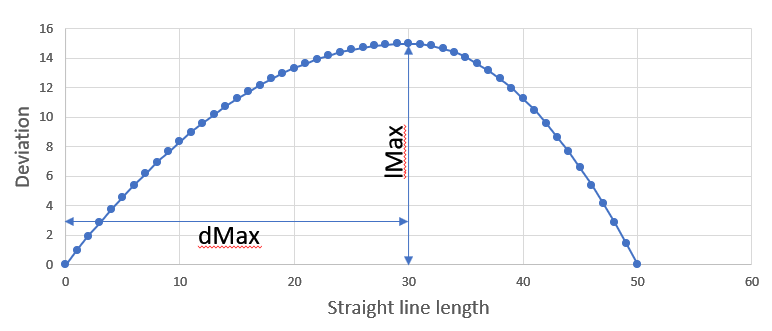
Note that if we pick dMax at mid point of the line (25 in this case), we'll get symmetric 'wings'.

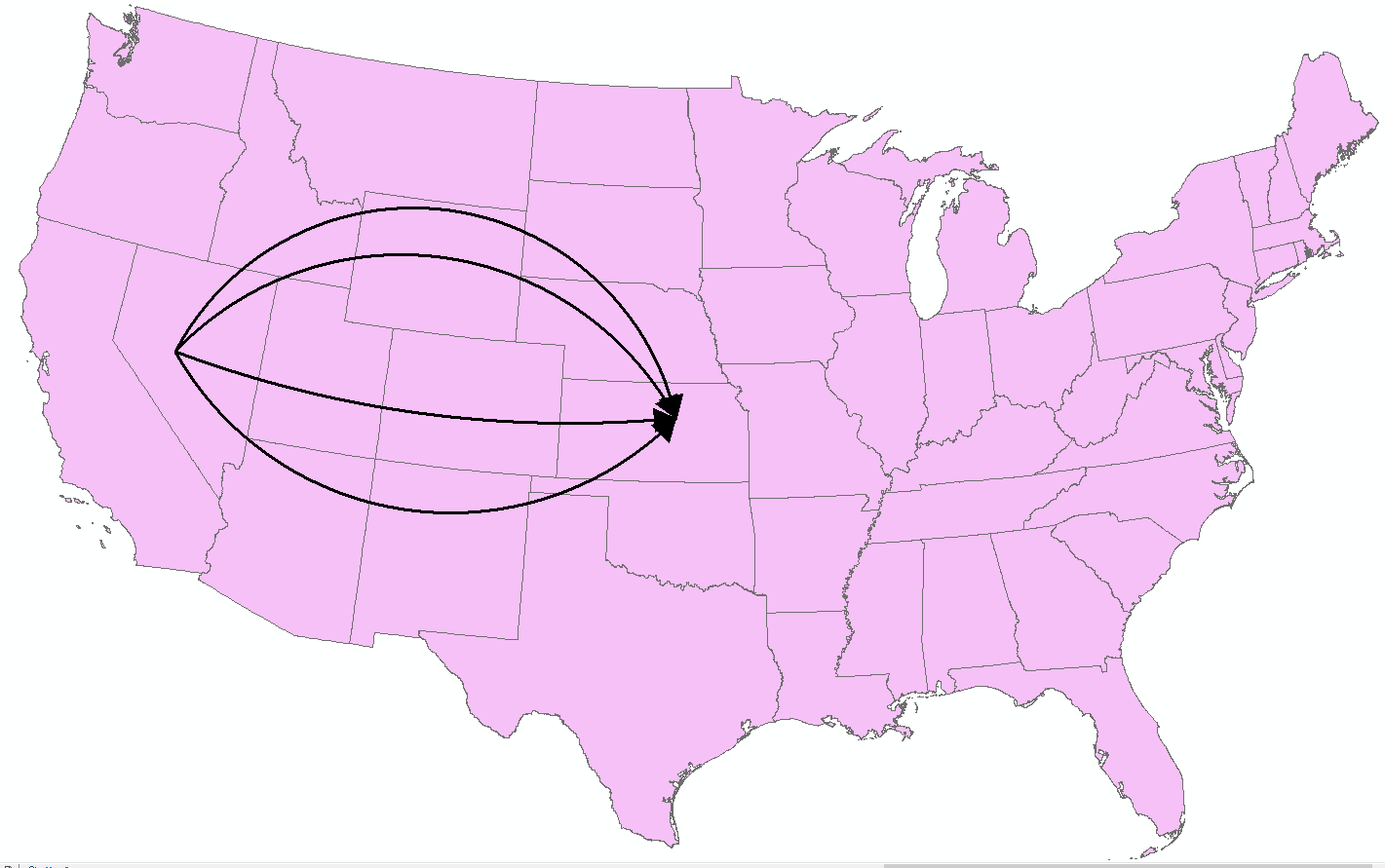
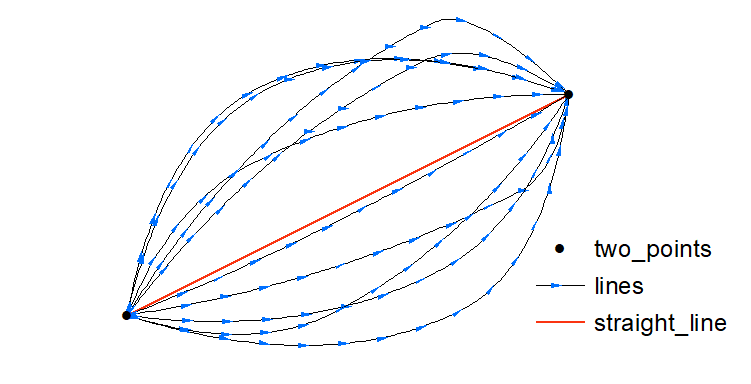
Output for 10 lines might look like this:

import numpy as np
import scipy, random

def RotateExtend(j):
    iDmax = random.randint(10, 90)  
    dMax = dAlong[iDmax]            # max deviation location (dMax)
    iLmax = random.randint(0, 30)   
    lMax = dAlong[iLmax]            # max deviation itself
    leftA = -lMax/dMax/dMax         # parabola coef left from dMax
    rightA = -lMax/(lenV-dMax)**2   # parabola coef right from dMax
##  length of perpendicular    
    sLength =np.array([lMax+(x-dMax)**2*[leftA,rightA][x>=dMax] for x in dAlong])
    i = j%2                         # left or right side of straight line
##  perpendicular end points
    sX = -dY*sLength/lenV
    sY = dX*sLength/lenV
    if i: sX+=DF_X;sY+=DF_Y
    else: sX = DF_X-sX;sY = DF_Y - sY
    points = arcpy.Array([arcpy.Point(x,y) for x,y in zip(sX,sY)])
    pLine = arcpy.Polyline(points)
    return pLine

infc = "two_points"
array = arcpy.da.FeatureClassToNumPyArray(infc, ["SHAPE@X","SHAPE@Y"])
XS,XE = array["SHAPE@X"]; dX = XE-XS
YS,YE = array["SHAPE@Y"]; dY = YE-YS
DF_X = np.linspace(XS,XE,100)
DF_Y = np.linspace(YS,YE,100)
dAlong = np.array([x[0] for x in scipy.spatial.distance.cdist(zip(DF_X,DF_Y),[[XS,YS]])])
lenV = dAlong[-1]
lines = map(RotateExtend,range(10))
outfc = arcpy.GetParameterAsText(0)
arcpy.management.CopyFeatures(lines,outfc)

If you need more than 10 polylines change this line of code accordingly:

lines = map(RotateExtend,range(**10**))