Projection planes that conform to the surface

Question

The ISEA projection and other global ones (see DGGS standard) showed that the technology exists: it is possible to use a "coarse cover of polygons" (typically triangles, squares or hexagons), to cover the Earth's crust, and use each polygon as a plane of projection. ISEA and other (ex. S2 Geometry) offers a continuous "projection cover", with no fitting problems — no border problem like in the old UTM systems.

So we can think of a "partial cover", without topological (spherical) compromise... The main question is "Is there a rigorous methodology (or software tools) for build it?"

Rationale: useful as "cover of projection planes" in a continental country such as Russia or Brazil. Each plane with the local mean altitude, and to build a hierarchical grid of equal-area cells.

---

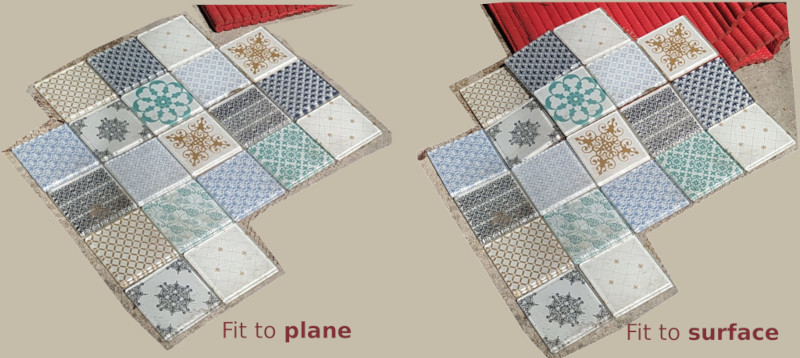
Illustrating with an intuitive home example:

ISEA and other projections are global, and are analogous to the "fit to plane" tiles (a set of planes that fit to sphere); I need a non-global and "fit to local surface" projection technology — each plane with a local median altitude.

---

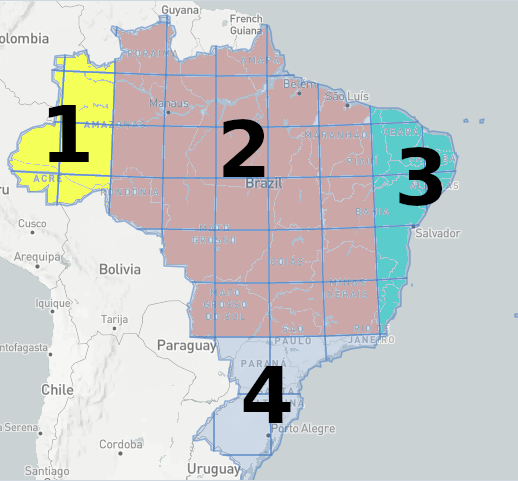
Bellow a concrete example, covering Brazil with 4 squares of ~3000 km of side: each with angular freedom to a local best fit to Brazilian's surface.
The labeled squares form 3 pairs connected by "hinges": 1-2; 2-3; 2-4.

PS: the supposed gain in accuracy with this technique could be compared to the Albers equal-area projection for full territory... perhaps no big gain, but I need to check.

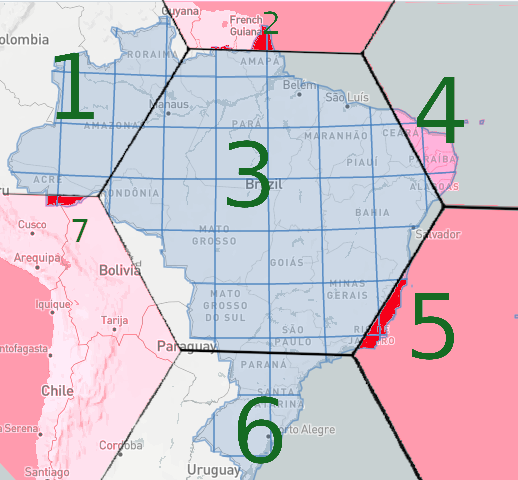
Bellow other example, covering Brazil with 7 hexagons: the main ones (labeled 1, 3, 4 and 6) each with angular freedom to a local best fit to Brazilian's surface, and the secondary ones (2, 5 and 7) where only a litle red portion are members of the country, with no border conflict with other hexagons. All connected hexagons has angular freedom: 1-3; 1-7; 3-2; 3-4; 3-5; 3-6.

---

Notes

• (edit after @mkennedy answer) in the LDP (low-distortion projections) jargon, we can say "map projection zones have zone overlap"... And LDP initiatives are not trying to solve the "span across multiple projection zones" problem.

• This question is not about arbitrary mosaic of projection zones, but about the use of a limited number of projection zones, that covers the country and have no border problem: a polygon can span across multiple projection zones, since they are into the country.

• Here a link to Wikipedia describing the classic overlapping UTM grid zones problem (and here a question about it). This question is about a technology that avoids the problem.

Answer 1

If I understand correctly what you wish to do, you should look up low-distortion projections (LDP). US National Geodetic Survey (NGS) is allowing states to propose some for the NSRS 2022 initiative (now scheduled to roll out in 2025 or so). An LDP is designed to take into account terrain so will have less overall distortion than an ellipsoid-surface-based projected coordinate reference system (ProjCRS). Dr. Michael Dennis has done several webinars on the planned CRS for 2022. Perhaps try this one first.

The ideal way to adjust a projection's parameters for local average elevation is to apply a scale factor. That parameter is common in many projections designed for large-scale / medium-scale like transverse Mercator, Lambert conformal conic, stereographic. These are all conformal. Not many of the equal area projections support a scale factor.

Another way to include an elevation adjustment was used by Minnesota for their LDP/county CRS. They adjusted the ellipsoid size/shape instead which is pretty difficult to deal with in ArcGIS, at least, and I suspect in some other GIS software.

Disclosure: I work for Esri.